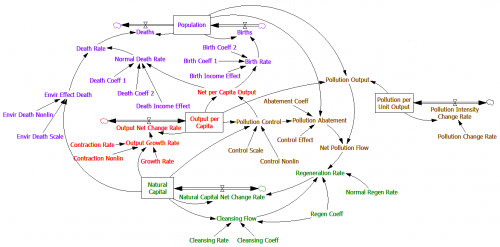
Wonderland model by Sanderson et al.; see Alexandra Milik, Alexia Prskawetz, Gustav Feichtinger, and Warren C. Sanderson, “Slow-fast Dynamics in Wonderland,” Environmental Modeling and Assessment 1 (1996) 3-17.
Here’s an excerpt from my 1998 critique of this model:
… The need for the upper/lower bound on natural capital highlights what I think is the problem with the model. The idea that the environment cannot be damaged if it’s initially pristine (NK=1) doesn’t really make sense. The upper and lower bounds to NK solve the problem computationally, but they don’t seem to have any real-world justification. I think it would be better to separate the damage process (from pollution) and the regeneration process. To do this, I think the concept of natural capital in the model needs to be clarified.
The logistic expression for equation 3 (natural capital change rate) suggests that natural capital means biomass of some sort. For real biomass, regeneration is logistic but damage is not, so the contributions of damage and regrowth should be additive, not multiplicative.
If, on the other hand, natural capital is meant to represent pollution, then an explicit pollution stock would be clearer. In this case, the emissions and uptake processes would still be additive, and uptake would be nonlinear but probably not logistic.
If you want both stock pollutants and biomass dynamics, then it seems that the environment in the model needs to be 2nd order.
On a related note, it seems to me that if natural capital = 0, output per capita ought to be zero, rather than contracting at a constant rate. Again, this depends on the meaning of natural capital. A formulation with an explicit capital stock and capital-labor-natural capital production function would be clearer, though more complex. This might also help to clarify the processes of pollution output vs. abatement.
Overall, I found the analysis of the model very interesting. Some of the fixes/clarifications/critiques I’ve listed above would make the slow-fast dynamics go away (mainly by changing the logistic behavior of natural capital, eliminating the attracting manifolds at NK = 0 and NK = 1). I’d like to see a model that fixes some of the problems I see, but preserves the interesting dynamics, esp. the 3 scenario trajectories.
This copy of the Wonderland model works only with Vensim DSS/Pro or the free Model Reader, as I created several macros to simplify the appearance of the S-shaped exponential functions and to prevent over/underflows. The model incorporates a modification of a fix suggested by the author that prevents Natural Capital from reaching exactly 0 or 1 due to roundoff error (see use of SINTEG in Natural Capital). The double-precision version of Vensim should work without the fix, though I have found that the model still tends to wander into the unrealistic trap of NK~=1.0. I can replicate the dream and nightmare scenarios from the source article (subject to the upper/lower bound parameter choice), though I can’t get an escape behavior without changing another parameter.
Wikipedia has a good article on the model and subsequent extensions.
This version includes an upgraded interface and slight technical improvements.
Wonderland3.vpm (published for Reader)
or
wonderland3.zip (mdl + cin files)