Category: Technical

Early warnings of catastrophe

Catastrophic Collapse Can Occur without Early Warning: Examples of Silent Catastrophes in Structured Ecological Models (PLOS ONE – open access)

Catastrophic and sudden collapses of ecosystems are sometimes preceded by early warning signals that potentially could be used to predict and prevent a forthcoming catastrophe. Universality of these early warning signals has been proposed, but no formal proof has been provided. Here, we show that in relatively simple ecological models the most commonly used early warning signals for a catastrophic collapse can be silent. We underpin the mathematical reason for this phenomenon, which involves the direction of the eigenvectors of the system. Our results demonstrate that claims on the universality of early warning signals are not correct, and that catastrophic collapses can occur without prior warning. In order to correctly predict a collapse and determine whether early warning signals precede the collapse, detailed knowledge of the mathematical structure of the approaching bifurcation is necessary. Unfortunately, such knowledge is often only obtained after the collapse has already occurred.

This is a third-order ecological model with juvenile and adult prey and a predator:

See my related blog post on the topic, in which I also mention a generic model of critical slowing down.

The model, with changes files (.cin) implementing some of the experiments: CatastropheWarning.zip

Social network valuation with logistic models

This is a logistic growth model for Facebook’s user base, with a very simple financial projection attached. It’s inspired by:

Quis pendit ipsa pretia: facebook valuation and diagnostic of a bubble based on nonlinear demographic dynamics

Peter Cauwels, Didier Sornette

We present a novel methodology to determine the fundamental value of firms in the social-networking sector based on two ingredients: (i) revenues and profits are inherently linked to its user basis through a direct channel that has no equivalent in other sectors; (ii) the growth of the number of users can be calibrated with standard logistic growth models and allows for reliable extrapolations of the size of the business at long time horizons. We illustrate the methodology with a detailed analysis of facebook, one of the biggest of the social-media giants. There is a clear signature of a change of regime that occurred in 2010 on the growth of the number of users, from a pure exponential behavior (a paradigm for unlimited growth) to a logistic function with asymptotic plateau (a paradigm for growth in competition). We consider three different scenarios, a base case, a high growth and an extreme growth scenario. Using a discount factor of 5%, a profit margin of 29% and 3.5 USD of revenues per user per year yields a value of facebook of 15.3 billion USD in the base case scenario, 20.2 billion USD in the high growth scenario and 32.9 billion USD in the extreme growth scenario. According to our methodology, this would imply that facebook would need to increase its profit per user before the IPO by a factor of 3 to 6 in the base case scenario, 2.5 to 5 in the high growth scenario and 1.5 to 3 in the extreme growth scenario in order to meet the current, widespread, high expectations. …

(via the arXiv blog)

This is not an exact replication of the model (though you can plug in the parameters from C&S’ paper to replicate their results). I used slightly different estimation methods, a generalization of the logistic (for saturation exponent <> 1), and variable revenues and interest rates in the projections (also optional).

This is a good illustration of how calibration payoffs work. The payoff in this model is actually a policy payoff, because the weighted sum-squared-error is calculated explicitly in the model. That makes it possible to generate Monte Carlo samples and filter them by SSE, and also makes it easier to estimate the scale and variation in the standard error of user base reports.

The model is connected to input data in a spreadsheet. Most is drawn from the paper, but I updated users and revenues with the latest estimates I could find.

A command script replicates optimization runs that fit the model to data for various values of the user carrying capacity K.

Note that there are two views, one for users, and one for financial projections.

See my accompanying blog post for some reflections on the outcome.

This model requires Vensim DSS, Pro, or the Model Reader. facebook 3.vpm or facebook3.zip (The .zip is probably easier if you have DSS or Pro and want to work with the supplementary control files.)

Update: I’ve added another set of models for Groupon: groupon 1.vpm, groupon 2.vpm and groupon.zip groupon3.zip

See my latest blog post for details.

 

Stochastic Processes

This model replicates a number of the stochastic processes from Dixit & Pindyck’s Investment Under Uncertainty. It includes Brownian motion (Wiener process), geometric Brownian motion, mean-reverting and jump processes, plus forecast confidence bounds for some variations.

Units balance, but after updating this model I’ve decided that there may be a conceptual issue, related to the interpretation of units in parameters of the Brownian process variants. This arises due to the fact that the parameter sigma represents the standard deviation at unit time, and that some of the derivations gloss over units associated with substitutions of dz=epsilon*SQRT(dt). I don’t think these are of practical importance, but will revisit the question in the future. This is what happens when you let economists get hold of engineers’ math. 🙂

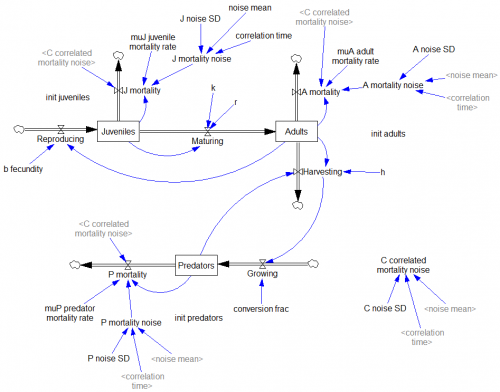
These structures would be handy if made into :MACRO:s for reuse.

stochastic processes 3.mdl (requires an advanced version of Vensim)

stochastic processes 3.vpm (published package; includes a sensitivity setup for varying NOISE SEED)

stochastic processes 3 PLE.mdl (Runs in PLE, omits only one equation of low importance)

Vensim Model Documentation Tool

Ignacio Martinez (U Chicago/Argonne, Vensim distributor, and all around nice guy) has developed a nifty tool that exploits Vensim’s open text file format and .dll to make very thorough, browsable model documentation.

It’s incredibly simple to use. Just unzip the archive, fire up the .exe, and point it at a model (.mdl format; it’ll also read some information out of an accompanying published .vpm, if there is one, but that’s not needed):

Continue reading “Vensim Model Documentation Tool”

Bifurcations from Strogatz’ Nonlinear Dynamics and Chaos

The following models are replicated from Steven Strogatz’ excellent text, Nonlinear Dynamics and Chaos.

These are just a few of the many models in the text. They illustrate bifurcations in one-dimensional systems (saddle node, transcritical, pitchfork) and one two-dimensional system (Hopf). The pitchfork bifurcation is closely related to the cusp catastrophe in the climate model recently posted.

Spiral from a point near the unstable fixed point at the origin to a stable limit cycle after a Hopf bifurcation (mu=.075, r0 = .025)

These are in support of an upcoming post on bifurcations and tipping points, so I won’t say more at the moment. I encourage you to read the book. If you replicate more of the models in it, I’d love to have copies here.

These are systems in normal form and therefore dimensionless and lacking in physical interpretation, though they certainly crop up in many real-world systems.

3-1 saddle node bifurcation.mdl

3-2 transcritical bifurcation.mdl

3-4 pitchfork bifurcation.mdl

8.2 Hopf bifurcation.mdl

Update: A related generic model illustrating critical slowing down:

critical slowing.mdl

Climate Catastrophe

This is an interesting, simple model of global ice age dynamics, from:

“A Catastrophe Model of the Paleoclimate”, Douglas R MacAyeal, Journal of Glaciology, Vol 24 No 90, 1979

It illustrates a pitchfork bifurcation as a slice through a cusp catastrophe. It’s conceptually related to earlier models by Budyko and Weertmans that demonstrated hysteresis in temperature and ice sheet dynamics.

The model is used qualitatively in the paper. I’ve assigned units of measure and parameter values that reveal the behavior of the catastrophe, but there’s no guarantee that they are physically realistic.

The .vpm package includes several .cin (changes) files that reproduce interesting tests on the model. The model runs in PLE, but you may want to use the Model Reader to access the .cin files in SyntheSim.

Catastrophe.vpm

 

Gumowski-Mira Attractor

I became aware of this neat model via the Vensim forum. I have no idea what the physical basis is, but the diverse and beautiful output it generates is quite amazing.

Interestingly, if you only looked at time series of this sequence, you’d probably never notice it.

This runs in any version of Vensim. gumowski mira.mdl

A Dynamic Synthesis of Basic Macroeconomic Theory

Model Name: A Dynamic Synthesis of Basic Macroeconomic Theory

Citation: Forrester, N.B. (1982) A Dynamic Synthesis of Basic Macroeconomic Theory: Implications for Stabilization Policy Analysis. PhD Dissertation, MIT Sloan School of Management.

Source: Provided by Nathan Forrester

Units balance: Yes, with 3 exceptions, evidently from the original publication

Format: Vensim

Notes: I mention this model in this article

A Dynamic Synthesis of Basic Macroeconomic Theory (Vensim .vpm)

Update: a newer version with improved diagrams and a control panel, plus changes files for a series of experiments with responses to negative demand shocks:

Download NFDis+TF-3.vpm or NFDis+TF-3.zip

The model runs in Vensim PLE, but you’ll need an advanced version to use the .cin and .cmd files included.

The Economic Long Wave

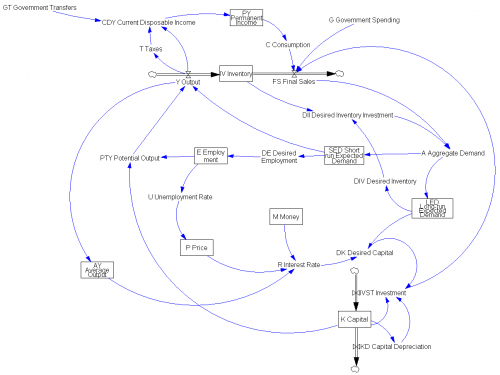
This is John Sterman’s model of long waves (long-duration economic cycles), driven by capital accumulation dynamics. This version is replicated from a JEBO article,

STERMAN, J. D. (1985) A Behavioral Model of the Economic Long Wave. Journal of Economic Behavior and Organization, 6, 17-53.

There’s some interesting related literature (including other economic models in this library). From Sterman’s publications list:

STERMAN, J. D. & MOSEKILDE, E. (1994) Business Cycles and Long Waves: A Behavioral, Disequilibrium Perspective. IN SEMMLER, W. (Ed.) Business Cycles: Theory and Empirical Methods. Boston, Kluwer Academic Publishers.

STERMAN, J. D. (1994) The Economic Long Wave: Theory and Evidence. IN SHIMADA, T. (Ed.) An Introduction to System Dynamics. Tokyo.

STERMAN, J. D. (2002) A Behavioral Model of the Economic Long Wave. IN EARL, P. E. (Ed.) The Legacy of Herbert Simon in Economic Analysis. Cheltenham, UK, Edward Elgar.

STERMAN, J. D. (1985) An Integrated Theory of the Economic Long Wave. Futures, 17, 104-131.

RASMUSSEN, S., MOSEKILDE, E. & STERMAN, J. D. (1985) Bifurcations and Chaotic Behavior in a Simple Model of the Economic Long Wave. System Dynamics Review, 1, 92-110.

STERMAN, J. D. (1983) The Long Wave. Science, 219, 1276.

KAMPMANN, C., HAXHOLDT, C., MOSEKILDE, E. & STERMAN, J. D. (1994) Entrainment in a Disaggregated Economic Long Wave Model. IN LEYDESDORFF, L. & VAN DEN BESSELAAR, P. (Eds.) Evolutionary Economics and Chaos Theory. London, Pinter.

MOSEKILDE, E., LARSEN, E. R., STERMAN, J. D. & THOMSEN, J. S. (1993) Mode Locking and Nonlinear Entrainment of Macroeconomic Cycles. IN DAY, R. & CHEN, P. (Eds.) Nonlinear Economics and Evolutionary Economics. New York, Oxford University Press.

MOSEKILDE, E., THOMSEN, J. S. & STERMAN, J. D. (1992) Nonlinear Interactions in the Economy. IN HAAG, G., MÜLLER, U. & TROITZSCH, K. (Eds.) Economic Evolution and Demographic Change. Berlin, Springer Verlag.

THOMSEN, J. S., MOSEKILDE, E. & STERMAN, J. D. (1991) Hyperchaotic Phenomena in Dynamic Decision Making. IN SINGH, M. G. & TRAVÉ-MASSUYÈS, L. (Eds.) Decision Support Systems and Qualitative Reasoning. Amsterdam, Elsevier Science Publishers.

THOMSEN, J. S., MOSEKILDE, E., LARSEN, E. R. & STERMAN, J. D. (1991) Mode-Locking and Chaos in a Periodically Driven Model of the Economic Long Wave. IN EBELING, W. (Ed.) Models of Self Organization in Complex Systems. Berlin, Akademie Verlag.

STERMAN, J. D. (1988) Nonlinear Dynamics in the World Economy: The Economic Long Wave. IN CHRISTIANSEN, P. & PARMENTIER, R. (Eds.) Structure, Coherence, and Chaos in Dynamical Systems. Manchester, Manchester University Press.

STERMAN, J. D. (1987) Debt, Default, and Long Waves: Is History Relevant? IN BOECKH, A. (Ed.) The Escalation in Debt and Disinflation: Prelude to Financial Mania and Crash? Montreal, BCA Publications.

STERMAN, J. D. (1987) An Integrated Theory of the Economic Long Wave. IN WANG, Q., SENGE, P., RICHARDSON, G. P. & MEADOWS, D. H. (Eds.) Theory and Application of System Dynamics. Beijing, New Times Press.

STERMAN, J. D. (1987) The Economic Long Wave: Theory and Evidence. IN VASKO, T. (Ed.) The Long Wave Debate. Berlin, Springer Verlag.

RASMUSSEN, S., MOSEKILDE, E. & STERMAN, J. D. (1987) Bifurcations and Chaotic Behavior in a Simple Model of the Economic Long Wave. IN WANG, Q., SENGE, P., RICHARDSON, G. P. & MEADOWS, D. H. (Eds.) Theory and Application of System Dynamics. Beijing, New Times Press.

And from Christian Kampmann,

“The Role of Prices in Long Wave Entrainment” (with C. Haxholdt, E. Mosekilde, and J.D. Sterman), International System Dynamics Conference, Stirling, U.K. and at the Spring 1994 ORSA/TIMS conference, Boston, MA. 1994.
“Disaggregating a simple model of the economic long wave” International Conference of the System Dynamics Society, Keystone, CO, 1985.
The long wave model was the guine pig for Kampmann’s interesting ’96 conference paper that combined a graph-theoretic identification of a set of feedback loops having independent gains with eigenvalue analysis,
Kampmann, Christian E.   Feedback Loop Gains and System Behavior
There also used to be a nifty long wave game, programmed on NEC minicomputers (32k memory?), but I’ve lost track of it. I’d be interested to here of a working version.